|
ABF305 Investment Management
Lecture 7
ABF305投资管理
第七讲
A review of the Put-Call parity theorem.
看跌期权评价理论的综述
This theorem states is that you can achieve the same investment outcome from a call option plus a treasury bill position that you can establish with a protective put.
这个定理的状态是,你可以实现相同的投资结果从一个看涨期权加上短期国库券的位置,你可以建立一个保护性看跌。
That being the case, the value of each position should be the same.
在这情况下,每个位置的值应是相同的。
Why is this important? To arrive at the put value for an option under the Black-Scholes option formula, it uses the put-call parity theorem.
为什么这很重要呢?认沽值到达Black-Scholes期权公式下的一个选项,它采用的把呼叫平价定理。
Let us see if we agree with this parity theorem.
We want a guaranteed investment outcome of $10,000 but we want an unlimited upside potential as well.
Choice (1) we buy a share and a protective put where the exercise price is $100. NB one option contract is for 100 shares. Our outcome: if the share price falls below $100, we exercise our put option, guaranteed income = $10,000. If the share price is above $100, we have an unlimited upside.
Choice (2) we buy a call option with the strike price $100 plus a zero coupon treasury bill where:
The face value of the T-Bill = the exercise price of the call.
The maturity of the T-Bill = the call option exercise date.
If the exercise price of the call is $100 and 1 contract = 100 shares, the face value of the T-Bill = $10,000.
If the stock price is ≤$100 we do not exercise our call. Our cash flow is the T-Bill = $10,000.
If the stock price is > $100 we exercise our option, so we get $10,000 from the T-Bill plus any upside above $100.
The outcomes are matched, so we agree with the theorem.
Hypothetically, we have proven that our investment outcome is equal so:
Where C = the call option
= the Face value of the T-Bill
= the exercise price of the call
S0 = Stock price
P = the protective put
The Put-Call parity theorem.
看跌期权评价理论
The put-call parity theorem relates the prices of put and call options as follows:
看跌期权评价理论涉及如下沽及认购期权的价格:
Where P is the put price, C is the call price, S0 is the stock price, X is the exercise price of both the call and the put options. PV(X) is the present value of a claim to X dollars to be paid at the expiration date of the options, and PV(dividends) is the present value of dividends to be paid before option expiration.
其中,P为认沽价,C为赎回价时,S0为股价,X是呼叫,而认沽期权的行使价。 PV(X)X美元的索赔支付期权的到期日期的现值和PV(股息)期权到期前支付股息的现值。
Two-State Option Pricing
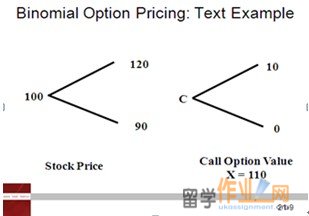
Example: Suppose that a stock is selling at $100 now and the price either increases (as denoted by ‘u’ for up) by a factor or 1.20 so it becomes $120, or it falls in value (as denoted by ‘d’ for down) by a factor of 0.90 at time T so the stock is now worth $90.
If a call option is written for the stock with an exercise price of $110, then with the movements in stock price, the payoff from the option will either be $10 or zero.
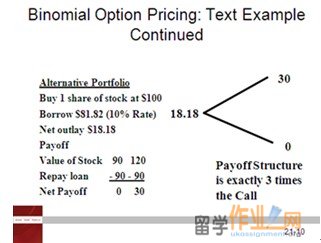
With this example, the payoff of the call is compared to a portfolio consisting of one share of the stock, and the borrowing of $81.82 at the interest rate of 10%.
If the borrowing of $81.82 is repaid after a year at the interest rate of 10%, it will be the equivalent of $90 at the end of the year.
If the stock price at the end of the year is either $90 or $120, then with the repayment of the loan, with our portfolio consisting of one share of stock and our borrowings, the portfolio payoff is either 0 or $30.
For our portfolio of a share and borrowings, since the initial share cost $100 and we borrowed $81.82, this means we needed to have a cash outlay of $18.18.
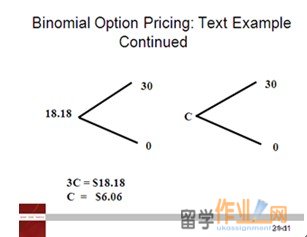
To demonstrate if the hedge ratio works:
证明是否对冲比率:
Write 3 options price 6.06 buy share $100, borrow $81.82 at 10%. Net cash flow = 0
At expiration: stock price is $90, options not exercised, repay loan $90. Net cash flow = 0
At expiration: stock price is $120, options exercised, pay $30 to holder, repay loan $90. Net cash flow = 0
There are six factors which affect the value of a call option:
1. Stock price
2. The volatility of the stock price
3. The time to expiration
4. The exercise price
5. The interest rate
6. The dividend rate of the stock.
To clarify:
The interest rate employed by the way is the risk-free interest rate but it is specifically the annualised continuously compounded rate on a safe asset with the same maturity as the expiration date of the option, which is to be distinguished from rf, the discrete period interest rate.
Why does the dividend rate and interest rate matter?
As the dividend payout increases, the value of the call option decreases because an increased dividend payout puts a drag on the rate of growth of the stock price.
The interest rate is employed to arrive at the present value for the exercise price. if interest rates rise, then the call option value will increase because higher interest rates reduce the present value of the exercise price.
Disadvantage of options:
In terms of their return on investment, options are very sensitivity to swings in the stock price. This sensitivity represents a disadvantage for an option.
We can demonstrate this sensitivity with an example.
Let us say you have $10,000 to invest and your investment choices are:
1. Buy shares in Firm X. Its share price is $100. You can thus buy 100 shares.
2. Buy options in Firm X. The call at-the-money option price for Firm X is $10 per share, and 1 call option contract is 100 shares. So each call option costs $1,000. You can thus buy 10 at-the-money contracts = $10,000
3. Your third strategy is to purchase 1 call option contract ($1,000 namely for 100 shares) and invest the remaining $9,000 in 6-month Treasury Bills which will earn 3% interest.
|





